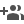Scientists have found a new way of spotting a property of topological space called topological invariant in quantum materials, that remains unchanged under continuous deformations or transformations.
Topological materials are at the forefront of next-gen technology—quantum computing, fault-tolerant electronics, and energy-efficient systems. But detecting their exotic properties has always been tricky. Topological invariance implies that if you can deform one shape into another without cutting or gluing, any topological invariant will be the same for both shapes. A popular analogy is the wada (or a donut) and a coffee cup. Since both the wada and the coffee cup have one hole, they are topologically equivalent. On the other hand, a wada and an idli are not, since you cannot continuously deform one into another as they have different numbers of holes. This idea of counting holes is key to understanding the hidden properties of exotic materials.
In certain materials like topological insulators and superconductors, strange things happen. Electrons behave differently depending on how the material is "shaped" at the quantum level. These shapes are defined not by their appearance, but by something deeper—topological invariants, such as winding numbers (in 1D systems) and Chern numbers (in 2D systems). These numbers are like hidden codes that determine how particles move through a material.
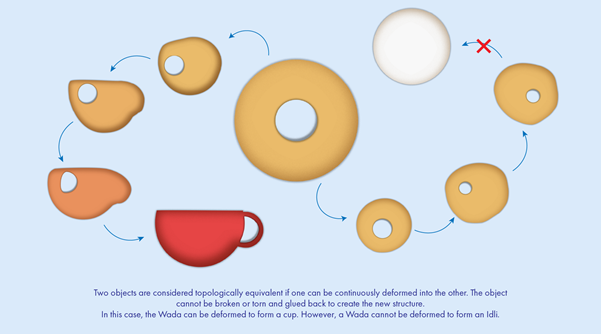
Fig 1: Representation of what is topological equivalence
A team from the Raman Research Institute, an autonomous institute of the Department of Science and Technology, found a new way to detect this hidden code using a property called the spectral function. This is something like a quantum fingerprint that reveals how energy and particles behave inside the material. Professor Dibyendu Roy and PhD researcher Kiran Babasaheb Estake have carried this out by analyzing the momentum-space spectral function (SPSF).
Traditionally, scientists used techniques like ARPES (Angle-Resolved Photoemission Spectroscopy) to study electron behaviour. The new research published in Physical Review B. showed that the same spectral function holds clues to the material’s hidden topology—a revolutionary way to “see” the structure without directly observing it.
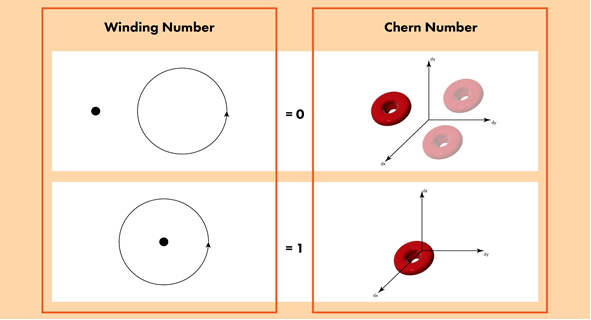
Fig 2: Representation of Winding number & Chern number
“The spectral function has been used since many years as an experimental tool to probe the physical quantities such as density of states and the dispersion relation of electrons in a system through ARPES. It was not seen as a tool to probe topology or topological aspects of an electronic system.” said Kiran Babasaheb Estake, PhD student in theoretical Physics at RRI and the lead author.
“We have demonstrated through various examples that the spectral function also contains signatures about the topology of a system,” he added.
The study potentially offers a universal tool to explore and classify topological materials, that could pave the way for new discoveries in condensed matter physics that could be useful for quantum computers, next generation electronics, and facilitate energy-efficiency.
Publication link: https://doi.org/10.1103/PhysRevB.111.085406
For further inquiries, contact at outreach[at]rrimail[dot]rri[dot]res[dot]in.